From a Physicist from Virginia University:
“I glanced at the page. It seemed interesting, but I see no mention of AD allowing Maxwell’s equations to remain invariant. Did I miss this??”
From the moment AD discards two frames in relative motion, the concept of “invariance” becomes meaningless. AD doesn’t use the Lorentz equations. AD replaces the Lorentz equations by Carezani’s equations. Equations (A) and (B) are replaced by equations (C) and (D).
(A) 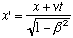 (B)
(B) 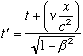
(C)  (D)
(D) 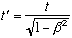
On the other hand, the question about “invariance” here is also a purely semantic question, regarding the following:
Maxwell’s equations at rest, in a system in motion with relative velocity to another system, is the same as saying that the phenomenon described by Maxwell’s equations is in motion with respect to the observer.
In AD, the phenomenon and observer form a physical system. We speak constantly about the Lorentz transformation, but in practice there is no transformation: The phenomenon is described by the phenomenon itself and the observer. In AD, the time t’ in equation (4) is reversible, that is to say:
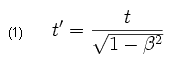
Here’s an example using Maxwell’s equations:
Taking the classical Maxwell’s equation in the form:
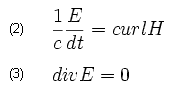
Writing the equation in general form and working it out we have
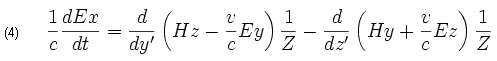
Where

It is deduced that an observer in motion, as well as observing an electric field E, will also observe a magnetic field H. The equations are “invariant,” but a different phenomenon appears when the observer, or the phenomenon, is in motion. Are the equations “invariant”?
The same application of AD when the “Maxwell Equation” (phenomenon described by ME) are in motion with respect to an observer, gives the following equation:
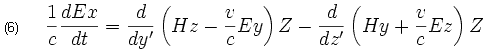
These two equations are conceptually equal with the exception that in the SR equation, the coefficient Z divides the equation and in AD, the coefficient multiplies the equation!