By Dr. Lucy Haye
Edited by David de Hilster
Even though we have previously explained Systems in Relative Motion and the derivation of the AD equations in many ways, it seems to us that the layman and probably many professionals are saturated with the Lorentz transformation and therefore the best explanation may be the following (see footnote 1):
A phenomenon happens at position A and an Observer at position B receives a signal from A
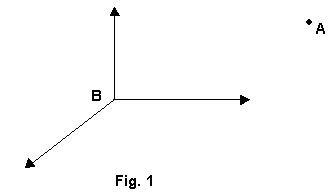
Fig. 1
Let us say that B has a System of Coordinates centered on it. Whether the phenomenon A is moving with respect to B, or B with respect to A, or both are moving simultaneously with respect to each other, is totally irrelevant to the Principle of Relativity. Light velocity is always constant, independent of both the Observer’s and the Phenomenon’s states of motion.
A simple question arises: Is there relativity to an Observer at B? Whoever is not saturated with the Lorentz transformation will answer the question with what makes sense: YES. Of course there is relativity between A and B, because the two fundamental rules of relativity are satisfied: inertial systems in relative motion and constant light velocity.
Those saturated with the Lorentz transformation will think in terms of two Observers, as if transmitting information between “two observers” is different from transmitting a signal between the Observer and the Phenomenon.
Two Observers in inertial systems in relative motion will measure the constancy of the light velocity and will accomplish with the Principle of Relativity exactly the same as is accomplished between an Observer at B and the Phenomenon at A. The information between the Phenomenon and the Observer yields relativity. What does the Lorentz transformation do?
Lorentz introduced a new System C in relative motion with respect to B but without motion with respect to A, that is, A is at rest in this new system that is artificially introduced.
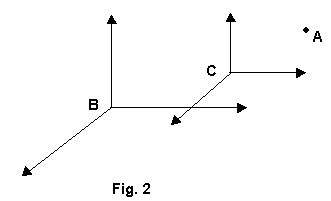
Fig. 2
Now it is very easy to show that this System of Coordinates (C) has no physical meaning, because when we move it to point A we do not lose the “relativity” between System B and System C or “System A.” See Fig. 3.
Fig. 3
But now, System C is totally irrelevant to the phenomenon at A – that is to say, it doesn’t have any physical meaning. System C also doesn’t have any meaning to System B, because an Observer at B will see the phenomenon directly, and System C is totally superfluous.
Using this clear explanation, we will show why the Lorentz transformation is physically wrong even though it is mathematically right.
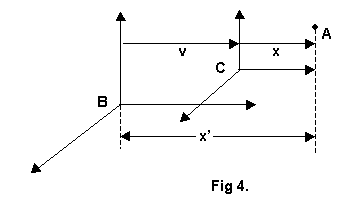
Fig 4.
In Fig. 4., x is a “constant distance” because A is at rest with respect to C. This means that the velocity that A has with respect to B is v – that is, the velocity of C with respect to B.
Now let us write the Lorentz equations:
(1) 
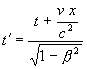(2)
We will write these equations as follows, where it is simple to see the variable separation
(3)
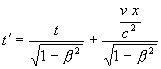(4)
It is very easy to see in equation (3) that x is divided by 
and this root decreases with the increasing velocity and consequently 
is larger than x. Yet this is not true: x is a constant distance because A is at rest in System C.
This increasing distance represents a velocity and this creates an artificial energy that later needs to be subtracted artificially using the Neutrino, postulated by Pauli to save SR’s failure to explain decay.
The same happens with time t in equation (4). The ‘local” time t in System B will not change because another System C is artificially introduced between A and B. t’ will change when measuring the phenomenon at A, but as a consequence, this will not introduce any change in the initial local time t.
SR is wrong because Einstein utilized the Lorentz transformation without any critical analysis. SR is physically wrong because the Lorentz transformation is also physically wrong.
What happens in Autodynamics?
In AD everything is flows nicely, makes sense, and represents the real physical world.
The AD equations are:
(5)
(6)
This is represented by Fig. (5), which is equivalent to Fig. 1.
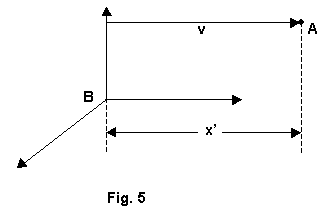
Fig. 5
But the “Two Systems in Relative Motion” constantly reverberate in the minds of people “saturated” with the Lorentz transformation.
OK. Let us go back to Fig.4, completing the picture in Fig. 6.
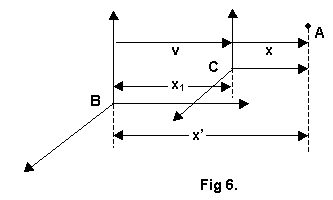
Fig 6.
In Autodynamics
x’ = x + x1 (7)
Now x1 in AD is
(8)
applying equation (5) to x1 because only System C is moving with respect to System B, but A is at rest with respect to C and we cannot apply equation (5).
Now
(9)
Autodynamics equation (9) is totally different from the Lorentz equations (1) or (3)
Autodynamics doesn’t increase the coordinate x, a constant distance, that must always stay constant and consequently does not introduce an ad hoc energy. No Neutrino is needed.
The following example is given using figure 15-1 and the equation 15.3 given by the Nobel Laureate Richard Feynman in his textbook on pages 15-2 and 15-3 respectively, in Volume 1 on “The Feynman Lectures on Physics” Addison-Wesley Publishing Company, Fifth Edition, July 1975.
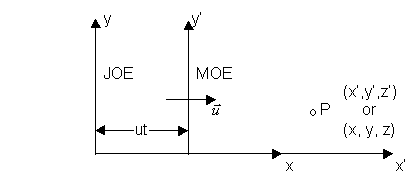
Fig. 15-1 Two coordinates systems in uniform relative motion along x-axes.
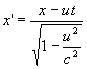(15.3)
We will calculate with the following values
x = 100 meter, u = 0.8 c meter/second, t = 10^-7 second
u = 0.8 * 300 000 000 = 240 000 000 meter/second
u t = 240 000 000 * 10^-7 = 24 meter
MOE starts traveling from JOE’s position at 0.8 of c for 10^-7 seconds. He will reach a distance of 126.666 meter which IS LARGER than the original distance x equal to 100 meters!
We can see this clearly by looking at equation (3) where
The constant distance x = 100 meters increase automatically to 166.666 meters.
MOE is traveling in the P direction but its distance from P increases!
MOE is traveling in the P direction and simultaneously he is away from it!
The Lorentz Transformation is amazing!
What happens in AD?.
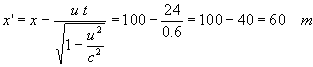
This makes sense!
It is evident that Lorentz failure is given by the fact that the coordinate of the point P is also divided by the root. This is false and absurd because it introduces variation on what is physically a constant.
Footnote 1 – This form was used for the first time by Carezani to explain this problem to Herman Leonard and was inspired by the latter through his questions prior to a photo session. Herman Leonard is a leading photographer in the USA, with collections at the Smithsonian Institution’s permanent collection on musical history. He is known for helping Yousuf Karsh photograph Einstein. Herman Leonard’s work can be found at:
http://www.hermanleonard.com and http://www.lpb.org/program/frame